

Coesite is a polymorph of silica, only stable at pressures in excess of ˜2.5 GPa at 500°C. At lower pressures it is thermodynamically unstable with respect to quartz. Before 1977 coesite was only known from meteorite impact craters, in which it was formed from quartz during the high pressures and temperatures generated by the impact and then quenched sufficiently rapidly to prevent reversion to quartz. More recently, preserved coesite has been found in deeply subducted crustal rocks in collision belts (see 1997 Annual Report); such occurrences provide definitive proof that the rocks in which it occurs have experienced depths of at least 100 km and have been exhumed subsequently under conditions that prevent the inversion to quartz (see 1997 Annual Report). Coesite can also be readily synthesised from quartz in the laboratory between pressures of 3 and 9 GPa at high temperatures; indeed the inversion between quartz and coesite is used as a standard calibration of pressure in the piston-cylinder apparatus, while its inversion at higher pressures to stishovite is used to calibrate multianvil presses in the 10 GPa pressure range.
A knowledge of the elastic properties of coesite is therefore essential for two reasons. Preservation of coesite in high-pressure rocks is dependent upon the exclusion of fluid which catalyses the inversion to quartz; this only occurs if the matrix of other minerals around the coesite remains uncracked. This in turn is determined by the elastic properties of the coesite and the matrix. Secondly, the compressional elastic constants are a derivative of volume with respect to pressure, so measurement of the elasticity and/or volume of coesite is necessary for more precise determination of the location of the transformations between coesite and the other silica polymorphs, which are determined in part by the associated volume change. Unfortunately, the data available in the literature on the elasticity of coesite derived from Brillouin spectroscopy measurements and from isothermal compression measurements made by X-ray diffraction are inconsistent. We therefore synthesised single-crystal samples of coesite at pressures from 3 to 7 GPa, and have undertaken new compression measurements by single-crystal X-ray diffraction in diamond-anvil cells with the aim of resolving this discrepancy.
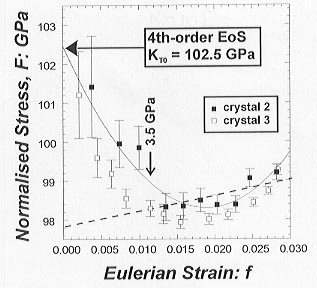
The results of our compression measurements are in excellent agreement with the literature data up to 4 GPa. The previously reported volume measurements up to 5.2 GPa then diverge to higher values than we measure in this pressure range, and are not consistent with the continuing smooth evolution of volume with pressure that we measure up to 9.6 GPa. Repeat measurements on further crystals, both from the same synthesis run in the multianvil press and on material synthesised in the piston-cylinder press, yield volume-pressure data that are identical within the small experimental uncertainties. However, despite the quality and the consistency of the data, the 3rd-order expansion of a finite-strain Equation of State, which is normally sufficient to describe the volume variation of minerals with pressure, does not fit the data. A plot of the normalised stress (F) against Eulerian strain (f) derived from the pressure-volume measurements (Fig. 3.1-1) reveals the nature of this anomalous behavior; the data plot on a parabola rather than the straight line corresponding to the 3rd-order EoS. Fitting the data with a fourth-order EoS yields a value of the second derivative of bulk modulus with respect to pressure, K² , which is large and positive, +0.65 GPa-1. Normally, K² has an implied negative value in a 3rd-order EoS, representing a slowing-down in the rate at which a material becomes stiffer upon compression. By contrast, coesite stiffens more rapidly as pressure increases, at least up to 10 GPa. Analysis of the compression of the individual unit-cell axes of the coesite suggests that this behavior is due to >60% of the volume compression being accommodated by the closing up of the feldspar-like "crankshaft chains" of SiO4 tetrahedra within the structure. As compression continues, the resistance of the Si-O-Si linkages to continuing reduction in angle must increase, resulting in this anomalous compression. The same compression mechanism operates in feldspars, but does not result in anomalous behavior. The reason appears to be that coesite, as a structure only stable at high pressures, is over-expanded at room pressure. Initial rapid compression occurs until a pressure >3.5 GPa at which point, with the structure compressed to a more "normal" configuration of Si-O-Si bonds, the compressional behavior also becomes more "normal" with a slightly positive slope in the f-F plot (Fig. 3.1-1).
|
Fig. 3.1-1: Single-crystal compression data for two crystals of coesite displayed as an f-F plot. Both datasets show strong curvature, and yield a room-pressure bulk modulus of ˜102 GPa for a 4th-order Equation of state (curved line for crystal 2). The straight dashed line is a 3rd-order EoS, which has an anomalously low value for KT0 ˜ 98 GPa. |
The identification of this anomalous behavior partially resolves the discrepancy between previous measurements of the elasticity of coesite. The value for the bulk modulus derived from our data is 102.5± 1 GPa for the 4th-order EoS, close to the value of KS0 = 109 GPa obtained by Brillouin spectroscopy (thermal expansion data is not available for coesite, so the KS0 to KT0 conversion cannot be made). By contrast the 3rd-order EoS fit to our data yields KT0 = 98 GPa, similar to previous determinations by X-ray diffraction which were additionally biased by the two errant data points at the highest pressures achieved.

Tel: +49-(0) 921 55 3700 / 3766, Fax: +49-(0) 921 55 3769, E-mail: bayerisches.geoinstitut(at)uni-bayreuth.de
 Previous page
Previous page