Previous page     Contents     Next page

Due to the difficulties in recovering samples of CaSiO₃ perovskite to ambient conditions, room-pressure measurements of the elasticity of this important lower-mantle mineral have yet to be made. Empirical systematic relationships between the measured elasticity of isostructural compounds and their physical and structural properties, such as molar volume or structural distortion, have therefore been used to predict the properties of such high pressure phases by extrapolation. On the basis of single-crystal compression data for CaTiO₃ and CaGeO₃ we previously proposed that a linear trend of bulk modulus with the inverse of the volume holds for Ca-perovskites. An improved test of the validity of this linear relationship could be obtained from precise elasticity data on Ca-perovskites with larger molar volumes than CaTiO₃. One candidate material is CaSnO₃ perovskite whose bulk modulus was previously measured at ambient conditions, but with an uncertainty of ˜10%.

We have measured elastic properties of CaSnO₃ perovskite by both ultrasonic interferometry and single-crystal X-ray diffraction at high pressures. We have used different data reduction techniques including reasonable estimated uncertainties to show that the final values of the elastic properties of CaSnO₃ perovskite are robust. The room-pressure bulk modulus of CaSnO₃ perovskite determined from our study is 10% higher than the previously determined value. Therefore the value of K_T0 of CaSnO₃ perovskite falls significantly above the linear trend of K_T0 with 1/V₀ (Fig. 3.1-6) previously thought to hold for Ca-bearing perovskites. Unfortunately, this means that doubt must be cast on the use of such a linear trend to estimate the bulk modulus of other Ca-perovskites, such as CaSiO₃, that are difficult to measure.

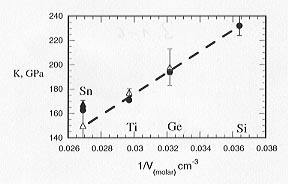Fig. 3.1-6: Bulk modulus of Ca-perovskites plotted against the inverse of molar volume. The B cation is indicated on the plot. The broken line is the previously proposed trend. The triangles are the ultrasonic data and the circles are the X-ray diffraction data. The solid symbols are from this study and the empty symbols are from the previous studies.

By contrast, we find a negative linear correlation (Fig. 3.1-7) between the shear moduli of Pbnm Ca-perovskites (CaSnO₃, CaTiO₃ and CaGeO₃) and their octahedral tilt angle 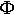. Extrapolation of this trend to 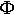= 0, corresponding to an undistorted cubic structure, yields a value of G₀˜150 GPa. Because cubic CaSiO₃ perovskite cannot deform macroscopically by shear without breaking the symmetry, we suggest that this value is a lower bound on the true shear modulus for this material. First-principles calculations for CaSiO₃ provided a value of G₀=165 GPa, consistent with this concept.

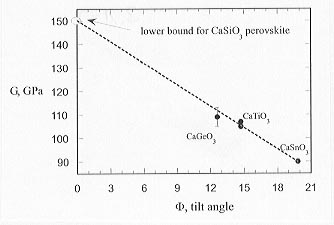Fig. 3.1-7: The variation of the shear modulus G0 for Ca-perovskites with the octahedral tilt angle 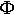. Extrapolation of the trend to 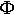=0 provides an estimated lower bound for the shear modulus of cubic Ca-perovskite such as CaSiO3.